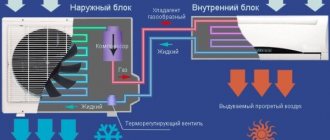
Loss of pressure in the system 02/24/2015 08:43
The resistance to the passage of air in a ventilation system is mainly determined by the speed of air movement in this system. As speed increases, resistance also increases. This phenomenon is called pressure loss. The static pressure created by the fan causes air movement in the ventilation system, which has a certain resistance. The higher the resistance of such a system, the lower the air flow moved by the fan or air handling unit. Calculation of friction losses for air in air ducts, as well as the resistance of network equipment (filter, silencer, heater, valve, etc.) can be made using the corresponding tables and diagrams indicated in the catalog. The total pressure drop can be calculated by summing the resistance values of all elements of the ventilation system.
Recommended air speed in air ducts:
| Side branches | 4,0 — 5,0 |
| Distribution ducts | 1,5 — 2,0 |
| Supply grilles near the ceiling | 1,0 – 3,0 |
| Exhaust grilles | 1,5 – 3,0 |
Determination of air speed in air ducts:
V= L / 3600*F (m/sec)
where L – air flow, m3/h; F – channel cross-sectional area, m2.
Recommendation 1: Pressure loss in a duct system can be reduced by increasing the cross-section of the ducts to ensure relatively uniform air velocities throughout the system. In the image we see how it is possible to ensure relatively uniform air speeds in a duct network with minimal pressure loss.
Recommendation 2. In systems with long air ducts and a large number of ventilation grilles, it is advisable to place the fan in the middle of the ventilation system. This solution has several advantages. On the one hand, pressure losses are reduced, and on the other hand, air ducts of a smaller cross-section can be used.
Example of calculation of a ventilation system: The calculation must begin by drawing up a sketch of the system indicating the locations of air ducts, ventilation grilles, fans, as well as the lengths of the air duct sections between the tees, then determine the air flow in each section of the network.
Let's find out the pressure loss for sections 1-6, using the graph of pressure loss in round air ducts, determine the required diameters of the air ducts and the pressure loss in them, provided that it is necessary to ensure the permissible air speed.
Section 1: air flow will be 220 m3/h. We assume the diameter of the air duct is 200 mm, the speed is 1.95 m/s, the pressure loss is 0.2 Pa/m x 15 m = 3 Pa (see the diagram for determining pressure loss in air ducts).
Section 2: let’s repeat the same calculations, not forgetting that the air flow through this section will already be 220+350=570 m3/h. We take the diameter of the air duct equal to 250 mm, speed – 3.23 m/s. The pressure loss will be 0.9 Pa/m x 20 m = 18 Pa.
Section 3: air flow through this section will be 1070 m3/h. We take the diameter of the air duct equal to 315 mm, the speed is 3.82 m/s. The pressure loss will be 1.1 Pa/m x 20= 22 Pa.
Section 4: air flow through this section will be 1570 m3/h. We take the diameter of the air duct equal to 315 mm, speed – 5.6 m/s. The pressure loss will be 2.3 Pa x 20 = 46 Pa.
Section 5: air flow through this section will be 1570 m3/h. We take the diameter of the air duct equal to 315 mm, speed 5.6 m/s. The pressure loss will be 2.3 Pa/m x 1= 2.3 Pa.
Section 6: air flow through this section will be 1570 m3/h. We take the diameter of the air duct equal to 315 mm, speed 5.6 m/s. The pressure loss will be 2.3 Pa x 10 = 23 Pa. The total pressure loss in the air ducts will be 114.3 Pa.
When the calculation of the last section is completed, it is necessary to determine the pressure loss in the network elements: in the CP 315/900 silencer (16 Pa) and in the KOM 315 check valve (22 Pa). We will also determine the pressure loss in the taps to the grilles (the total resistance of 4 taps will be 8 Pa).
Determination of pressure loss at bends of air ducts
The graph allows you to determine the pressure loss in the outlet based on the bend angle, diameter and air flow.
Example. Let us determine the pressure loss for a 90° outlet with a diameter of 250 mm at an air flow of 500 m3/h. To do this, we find the intersection of the vertical line corresponding to our air flow with an inclined line characterizing the diameter of 250 mm, and on the vertical line on the left for a 90° outlet we find the value of pressure loss, which is 2 Pa.
We accept for installation ceiling diffusers of the PF series, the resistance of which, according to the schedule, will be 26 Pa.
Now let’s sum up all the pressure loss values for straight sections of air ducts, network elements, bends and grilles. The desired value is 186.3 Pa.
We calculated the system and determined that we need a fan that removes 1570 m3/h of air with a network resistance of 186.3 Pa. Taking into account the characteristics required for the operation of the system, we will be satisfied with the fan; the characteristics required for the operation of the system will suit us with the VENTS VKMS 315 fan.
Calculation of local resistance in ventilation systems
2012-02-15
(0) (17195) (0)
Published in SOK magazine No. 2 | 2012
Rubric:
- Ventilation/conditioning
Tags:
- Airwell
- AERECO
- Ventilation
As is known [1], during the movement of air flow in ventilation and air conditioning systems (V and AC), in addition to pressure losses due to friction, losses on local resistances - shaped parts of air ducts, air distributors and network equipment - play a large role.
| Such losses are proportional to the dynamic pressure pd = ρv2/2, where ρ is the air density, equal to approximately 1.2 kg/m3 at a temperature of about +20 °C, and v is its speed [m/s], usually behind resistance. Proportionality coefficients ζ, called local resistance coefficients (KMC), for various elements of B and HF systems are usually determined from tables available, in particular, in [2] and in a number of other sources. The greatest difficulty in this case is most often the search for KMS for tees or branch assemblies, since in this case it is necessary to take into account the type of tee (for passage or for branch) and the mode of air movement (discharge or suction), as well as the ratio of air flow in the branch to flow rate in the barrel Loʹ = Lo/Lc and cross-sectional area of the passage to the cross-sectional area of the barrel fnʹ = fn/fc. For tees during suction, it is also necessary to take into account the ratio of the cross-sectional area of the branch to the cross-sectional area of the trunk foʹ = fo/fc. In the manual [2], the relevant data is given in table. 22.36–22.40. However, at high relative flow rates in the branch, the RMCs change very sharply, therefore, in this area, the tables under consideration are manually interpolated with difficulty and with a significant error. In addition, in the case of using MS Excel spreadsheets, it is again desirable to have formulas for directly calculating the CMR through the ratio of flow rates and sections. Moreover, such formulas should, on the one hand, be quite simple and convenient for mass design and use in the educational process, but, at the same time, should not give an error exceeding the usual accuracy of engineering calculations. Previously, a similar problem was solved by the author in relation to resistances encountered in water heating systems [3]. Let us now consider this issue for mechanical systems B and HF. Below are the results of data approximation [2] for unified tees (branch nodes) per passage. The general form of the dependencies was chosen based on physical considerations, taking into account the convenience of using the resulting expressions while ensuring an acceptable deviation from the tabular data: ❏ for supply tees, with Loʹ ≤ 0.7 and fnʹ ≥ 0.5: and with Loʹ ≤ 0.4 you can use a simplified formula: ❏ for exhaust tees: It is easy to notice that the relative area of the passage fnʹ during discharge or, respectively, the branch foʹ during suction affects the CMS in the same way, namely, with an increase in fnʹ or foʹ the resistance will decrease, and the numerical coefficient for the specified parameters in all given formulas is the same, namely (–0.25). In addition, for both supply and exhaust tees, when the air flow rate in the branch changes, the relative minimum KMS occurs at the same level Loʹ = 0.2. These circumstances indicate that the obtained expressions, despite their simplicity, sufficiently reflect the general physical laws underlying the influence of the studied parameters on pressure losses in tees of any type. In particular, the larger fnʹ or foʹ, i.e. the closer they are to unity, the less the flow structure changes when passing resistance, and therefore the less the CMR. For the Loʹ value the dependence is more complex, but here it will be common to both modes of air movement. An idea of the degree of correspondence between the found relationships and the initial CMR values is given in Fig. 1, which shows the results of processing Table 22.37 for KMS standardized tees (branch assemblies) for the passage of round and rectangular cross-sections during injection. Approximately the same picture is obtained for the approximation of the table. 22.38 using formula (3). Note that, although in the latter case we are talking about a circular cross-section, it is easy to see that expression (3) quite successfully describes the data in the table. 22.39, already related to rectangular nodes. The error of formulas for CMS is generally 5–10% (maximum up to 15%). Slightly higher deviations may be given by expression (3) for tees during suction, but even here this can be considered satisfactory, taking into account the complexity of changing the resistance in such elements. In any case, the nature of the dependence of the IMR on the factors influencing it is reflected very well here. In this case, the obtained relationships do not require any other initial data other than those already available in the aerodynamic calculation table. In fact, it must explicitly indicate both the air flow rates and the cross sections in the current and adjacent sections included in the listed formulas. This especially simplifies calculations when using MS Excel spreadsheets. At the same time, the formulas given in this work are very simple, clear and easily accessible for engineering calculations, especially in MS Excel, as well as in the educational process. Their use makes it possible to abandon the interpolation of tables while maintaining the accuracy required for engineering calculations, and to directly calculate the CMC of tees per passage for a wide variety of cross-sectional ratios and air flow rates in the trunk and branches. This is quite enough for the design of V and HF systems in most residential and public buildings. 1. A.D. Altshul, L.S. Zhivotovsky, L.P. Ivanov. Hydraulics and aerodynamics. - M.: Stroyizdat, 1987. 2. Designer's Handbook. Internal sanitary installations. Part 3. Ventilation and air conditioning. Book 2 / Ed. N.N. Pavlova and Yu.I. Schiller. - M.: Stroyizdat, 1992. 3. O.D. Samarin. On the calculation of pressure losses in elements of water heating systems // Journal S.O.K., No. 2/2007. |
(0) (17195) (0)
Send to a friend
tweet
Determination of pressure losses in air ducts.
Determination of pressure loss in a check valve.
Selection of the required fan.
Determination of pressure loss in silencers.
Determination of pressure losses at bends of air ducts.
Determination of pressure loss in diffusers.
Where to start?
Diagram of pressure loss for each meter of duct.
Very often you have to deal with fairly simple ventilation schemes, in which there is an air duct of the same diameter and there is no additional equipment. Such circuits are calculated quite simply, but what if the circuit is complex with many branches? According to the method for calculating pressure losses in air ducts, which is set out in many reference publications, it is necessary to determine the longest branch of the system or the branch with the greatest resistance. It is rarely possible to determine resistance by eye, so it is customary to carry out calculations based on the longest branch. After this, using the air flow rates indicated on the diagram, the entire branch is divided into sections according to this criterion. As a rule, costs change after branches (tees) and when dividing it is best to focus on them. There are other options, for example, supply or exhaust grilles built directly into the main air duct. If this is not shown in the diagram, but such a grid is available, you will need to calculate the flow rate after it. Areas are numbered starting from the one furthest from the fan.